
Technological
Communication
Biosci. Biotech. Res. Comm. 10(2): 297-310 (2017)
Novel fuzzy optimal controller based on STATCOM to
damp SSR oscillations in series compensated systems
Zahra Rahimkhani
Department of Computer Science, Sarvestan Branch, Islamic Azad University, Sarvestan, Iran
ABSTRACT
Series compensation of transmission lines connected to turbo generators can result in Sub Synchronous Resonance
(SSR) leading to adverse torsional oscillations. SSR leads to turbine-generator shaft failure and system instability. The
use of Flexible AC Transmission System (FACTS) controllers such as Static Synchronous Compensator (STATCOM)
are increasing in the network for enhancing power transfer capability, dynamic voltage support and also damping of
power oscillations. STATCOM is one of the most versatile exible ac transmission system (FACTS) controllers which
controls the reactive power ows in transmission lines originating from a substation while controlling the sending
end bus voltage. This paper reports the analysis and study of novel supplementary sub synchronous damping control-
ler (SSDC) for STATCOM which is capable of damping out sub synchronous Resonance (SSR) oscillations in power
system with series compensated transmission lines. Proposed SSDC for STATCOM is designed based on a hybrid fuzzy
optimal controller to damp all SSR torsional oscillations. Simulation results which are obtained by MATLAB, verify
the effectiveness of proposed technique and its control strategy for enhancing stability.
KEY WORDS: STATCOM, OSCILLATION DAMPING, FUZZY CONTROLLER, OPTIMAL CONTROLLER, SUB SYNCHRONOUS RESONANCE
297
ARTICLE INFORMATION:
*Corresponding Author: isi.rahimkhani@gmail.com
Received 12
th
March, 2017
Accepted after revision 28
th
June, 2017
BBRC Print ISSN: 0974-6455
Online ISSN: 2321-4007 CODEN: USA BBRCBA
Thomson Reuters ISI ESC and Crossref Indexed Journal
NAAS Journal Score 2017: 4.31 Cosmos IF : 4.006
© A Society of Science and Nature Publication, 2017. All rights
reserved.
Online Contents Available at: http//www.bbrc.in/
INTRODUCTION
Series capacitors have extensively been used as a very
effective means of increasing the power transfer capa-
bility of transmission lines and improving transient
and steady state stability limits of power systems (IEEE
SSR Working Group 1985). These improvements are
done by compensating reactance of the transmission
lines. Besides of having remarkable pro ts for this kind
of compensation for transmission line, the risk of SSR

298 NOVEL FUZZY OPTIMAL CONTROLLER BASED ON STATCOM BIOSCIENCE BIOTECHNOLOGY RESEARCH COMMUNICATIONS
Zahra Rahimkhani
could also be brought to the power system which could
cause severe damages to the shaft of the generator unit
(Xie et al 2012 and Zhu et al 2014).
SSR is a condition of an electrical power system
where electrical networks exchange energy with the
mechanical system of the generator at frequencies less
than the nominal frequency of the transmission line. At
this situation, the turbine-generator oscillates at a fre-
quency corresponding to the torsional mode frequency.
The torsional oscillations may raise and result in the
failure of the turbine shaft (Orman et al 2012).
Numerous papers have been published about damp-
ing the SSR phenomenon, (Fouad et al 1978, Iravani
et al 1994), frequency scanning method (Framer et al
1985, Rana et al 2009), time domain simulation (Zhu
et al 1995) and using exible AC transmission systems
(FACTSs) controllers such as the static synchronous
series compensator (SSSC) Padiyar et al (2006) (Bon-
giorno et al (2008), the uni ed power ow controller
(UPFC) (Bo et al (2002), the thyristor controlled series
capacitor (TCSC) (Pilotto et al (2003)) and high voltage
direct current (HVDC) transmission systems (Jiang et al
(2005) have been applied to prevent the SSR in power
systems.
Rotor oscillations of generator at a torsion mode fre-
quency, (fm) induce armature voltage components at
frequencies (fern) given by:
When the sub synchronous component term is close
to electrical resonant frequency, the sub synchronous
torques produced by sub synchronous voltage compo-
nents can be sustained. This interplay between electrical
and mechanical systems is termed as torsional oscilla-
tions ( Xie et al (2012).
As a new type of reactive power compensation FACTS
device, STATCOM has a fast and smooth control per-
formance. By applying appropriate control strategies,
STATCOM could be used to damp power frequency oscil-
lation, enhancing power transfer and voltage stability.
In this paper, the damping of torsional oscillations
using STATCOM has been studied and novel supplemen-
tary sub synchronous damping controller based on fuzzy
optimal technique is proposed. Optimal control method
which is used along fuzzy damping controller will be
designed based on linear quadratic regulator (LQR) that
minimizes the cost function in order to achieve the
optimal tradeoff between the use of control effort, the
magnitude and the speed of response. Also it guaran-
tees a stable control system. The Fuzzy logic is used to
design of control system in outer loops of controller and
designed supplementary controller for damping oscilla-
tion in STATCOM. Simulation results which is obtained
by MATLAB, veri es the effectiveness of the STATCOM
and its control strategy for damping SSR oscillations.
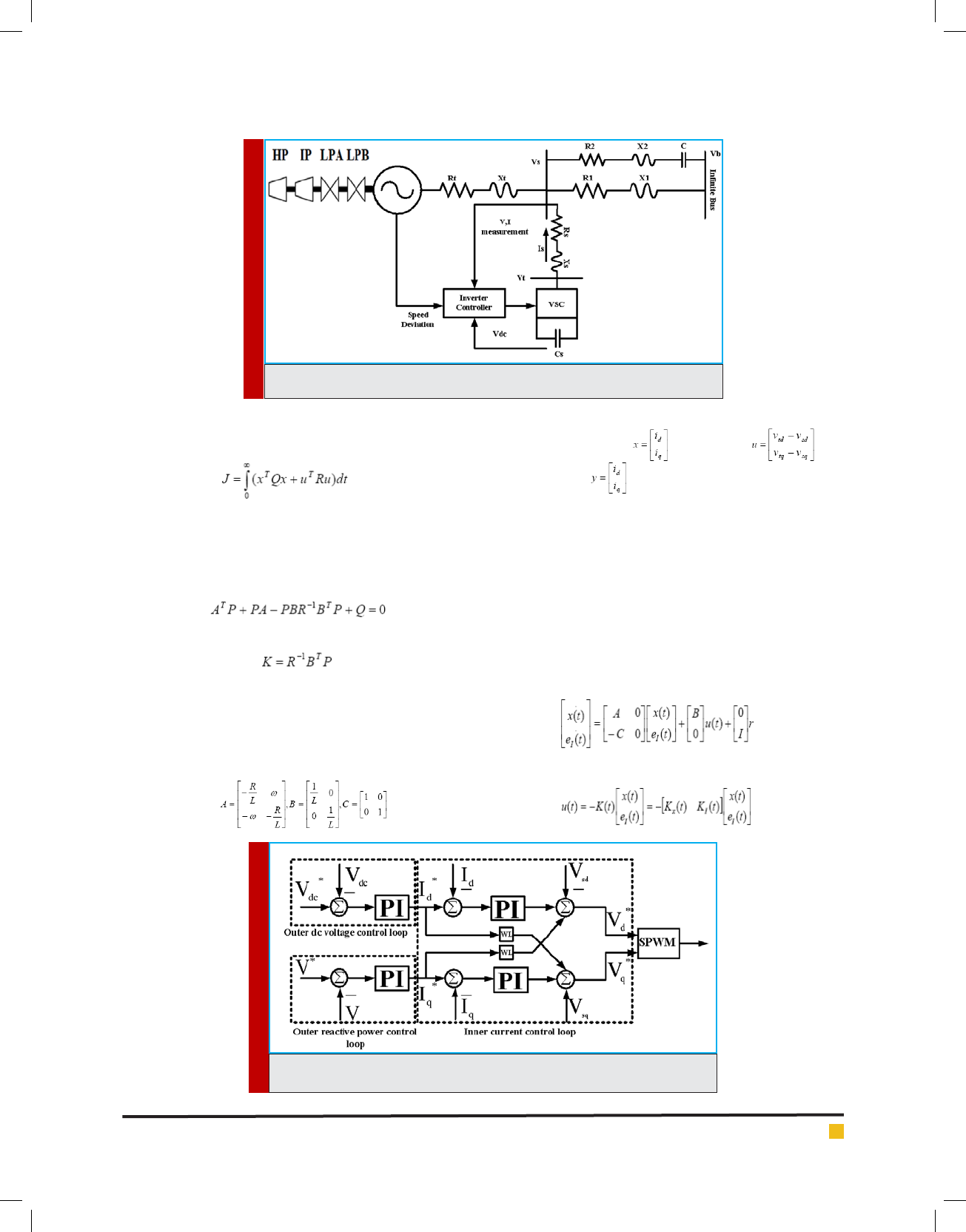
DYNAMIC MODELLING OF STATCOM
The system considered is an IEEE benchmark used to
study subsynchronous resonance. The modeling aspects
of the electromechanical system are given in detail in
reference. This system is shown in Fig.1.
One convenient method for studying balanced three-
phase system (especially in synchronous machine
problems) is to convert the three phase voltages and
currents into synchronous rotating frame by abc/dq
transformation. The bene ts of such arrangement are:
the control problem is greatly simpli ed because the
system variables become DC values under balanced
condition; multiple control variables are decoupled so
that the use of classic control method is possible, and
even more physical meaning for each control variable can
be acquired. Equations (2) to (4) give the mathematical
expression of the STATCOM shown in Fig 1.
Fig. 2 illustrates the detailed control block diagram
of STATCOM according to dynamic equations (Xin et al
2009 and Yidan et al 2011).
OPTIMAL CONTROLLER BASED ON LQR
The theory of optimal control is concerned with operat-
ing a dynamic system at minimum cost. The case where
the system dynamics are described by a set of linear dif-
ferential equations and the cost is described by a quad-
ratic function is called the LQ problem. One of the main
results in the theory is that the solution is provided by
the linear-quadratic regulator (LQR), a feedback control-
ler whose equations are given below.
This method determines the feedback gain matrix
that minimizes the cost function in order to achieve the
optimal tradeoff between the use of control effort, the
magnitude and the speed of response. In addition, this
method guarantees a stable control system.
Given a linear system:
Where x (t) are the system’s states, u(t) is the system
input and y(t) is the output. The objective is to design a
(1)
(2)
(3)
(4)
(5)
BIOSCIENCE BIOTECHNOLOGY RESEARCH COMMUNICATIONS NOVEL FUZZY OPTIMAL CONTROLLER BASED ON STATCOM 299
Zahra Rahimkhani
feedback u(t) = -Kx(t) such that the cost function (4) can
be minimized:
The weighting matrices Q and R are positive semi-
de nite. They control how much effort should be put
on the controller. The feedback gain K is obtained by
getting matrix P rst via solving the Riccati equation:
Therefore,
When the feedback gain K is obtained, the LQR con-
troller can be easily designed to make the states approach
zeros optimally.
Writing equations (2), (3) and (4) in the state space
format as (5), the corresponding matrix can be found as:
Where, the states
, the inputs and
the output are .
Since the LQR controller is designed to drive the
states to zero. This is very restrictive and not suitable
for solving tracking system problem. In the STATCOM
control, line currents are to be followed. Therefore,
alteration must be applied to the LQR controller in order
to drive the current errors, instead of the currents, to
zero. To achieve zero steady state errors, an integrator
is inserted in the control loop and the original system
is augmented to include the errors as new system states
(Xie et al (2012).
In equation (8),
(6)
FIGURE 1. Single line diagram of IEEE benchmark test system
FIGURE 2. The detailed control block diagram of STATCOM.
(7)
(8)
(9)
(10)
300 NOVEL FUZZY OPTIMAL CONTROLLER BASED ON STATCOM BIOSCIENCE BIOTECHNOLOGY RESEARCH COMMUNICATIONS
Zahra Rahimkhani
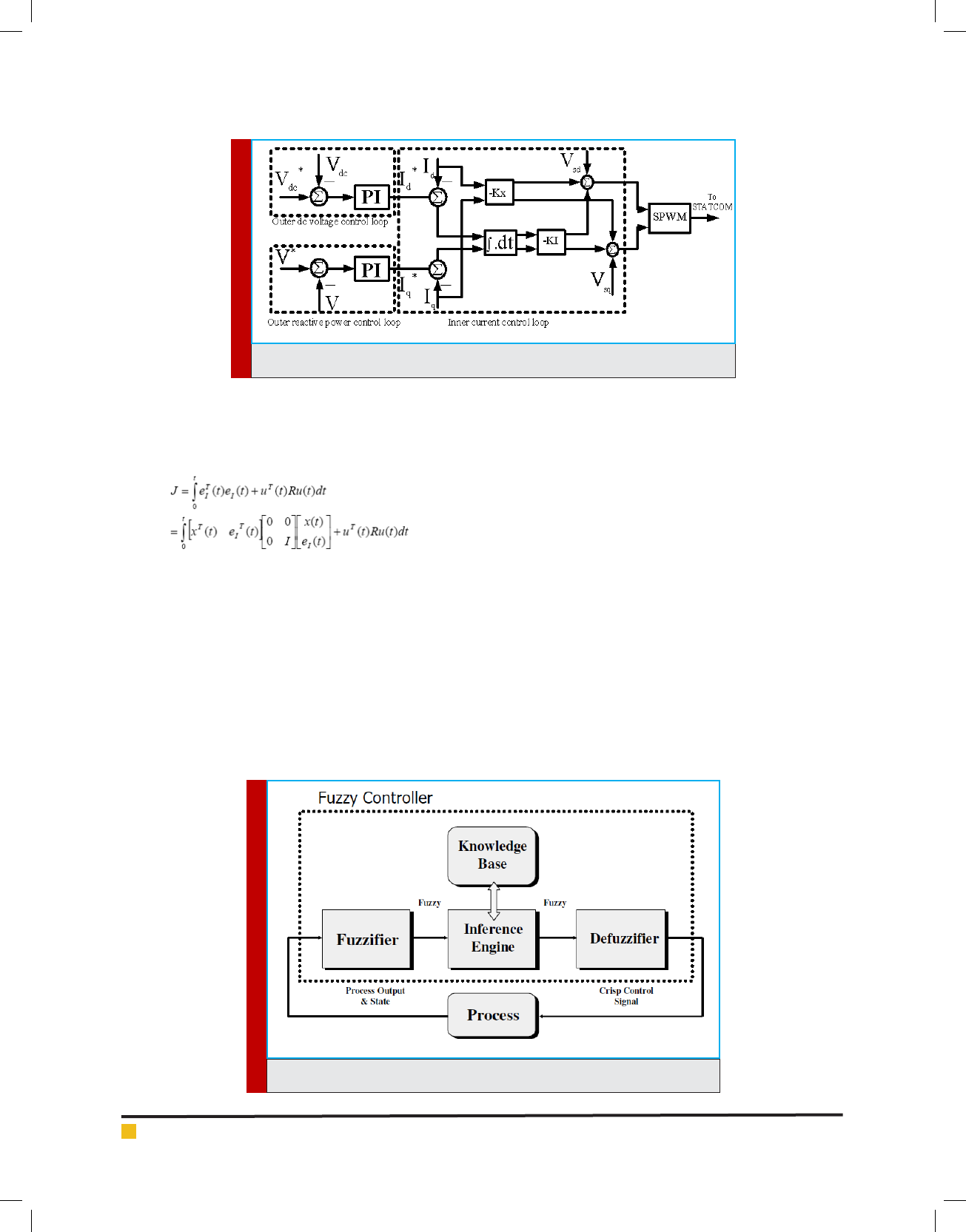
Rewrite the cost function in format of (10), it shows
that the new LQR regulator is aimed in minimize the
errors.
The control block diagram of the LQR current con-
trol loop which is proposed for STATCOM is shown
in Fig.3.
CONTROLLER DESIGN BASED ON FUZZY LOGIC
Fuzzy control is a control method based on fuzzy logic.
Just as fuzzy logic can be described simply as “comput-
ing with words rather than numbers”; fuzzy control can
be described simply as “control with sentences rather
than equations” (Jang et al 2010). A fuzzy controller can
include empirical rules, and that is especially useful in
operator controlled plants.Different parts of fuzzy con-
troller are shown in Fig.4.
The major components of a typical fuzzy controller
are fuzzi cation, fuzzy rule base, fuzzy inference, and
defuzzi cation. Fuzzi cation is the process of decom-
posing a system input and/or output into one or more
fuzzy sets. A fuzzy set is represented by a membership
function de ned on the universe of discourse. Fuzzy
rules represent the control strategy. They are linguistic
if-then statements involving fuzzy set, fuzzy logic, and
fuzzy inference (Single et al 2013).
Fuzzy rules play a key role in representing expert
control knowledge and experience and in linking the
input variables of fuzzy controllers to output variable
(or variables). Fuzzy inference is used in a fuzzy rule to
determine the rule outcome from the given rule input
information (Mon et al (2013).
FIGURE 3. The control block diagram of the LQR current control loop.
(11)
FIGURE 4. Common Structure of Fuzzy Controller
Zahra Rahimkhani
BIOSCIENCE BIOTECHNOLOGY RESEARCH COMMUNICATIONS NOVEL FUZZY OPTIMAL CONTROLLER BASED ON STATCOM 301
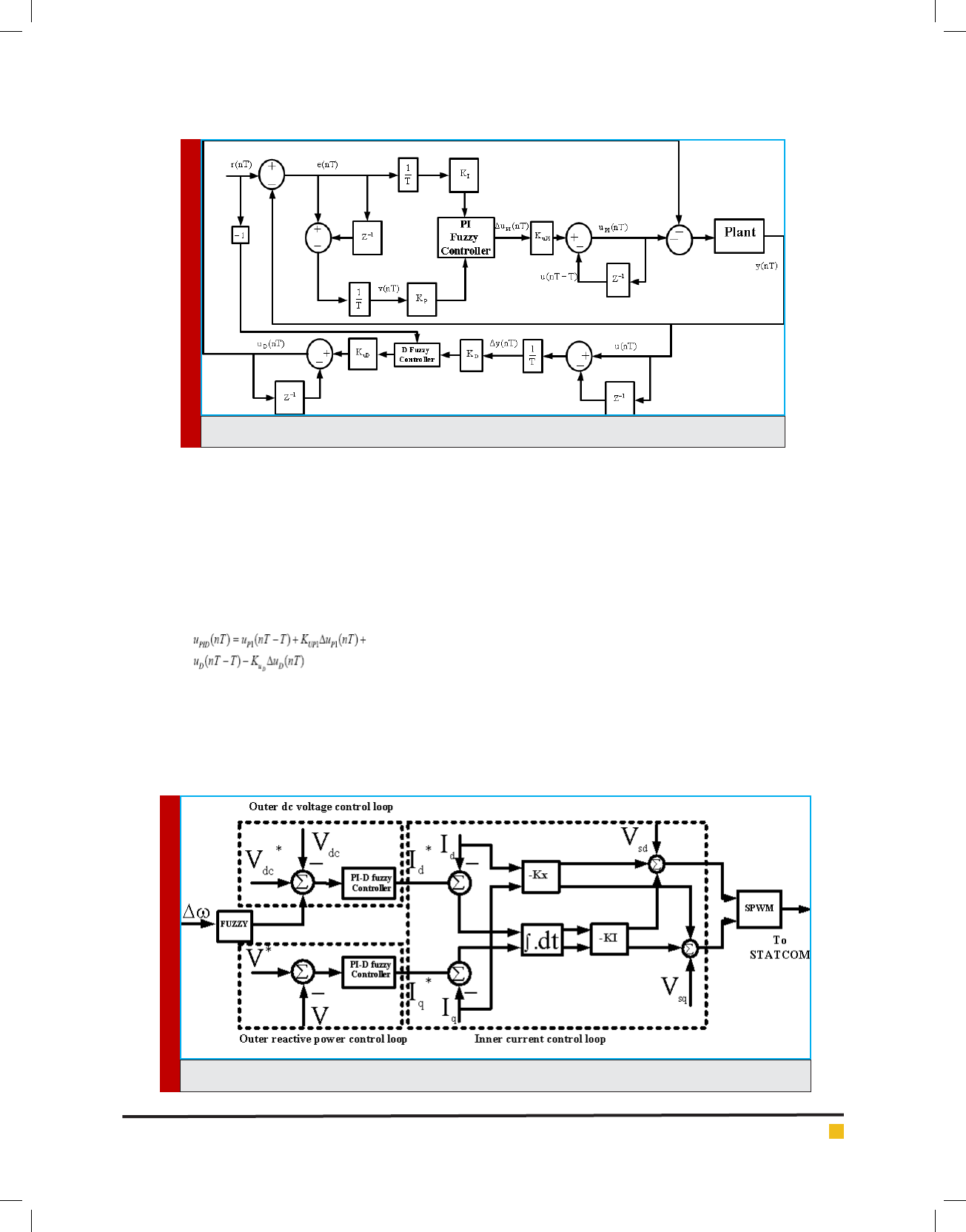
In this article a fuzzy PI-D control units is used with
the STATCOM control circuit. This controller is shown
in Fig.5.in this gure the derivation of the fuzzy control
law is performed in two steps: one for the output of the
fuzzy PI controller and the other for the output of the
fuzzy D controller. The nal control law combines these
two individual control laws together in an appropriate
way. As follow:
PROPOSED OPTIMAL PI-D FUZZY CONTROLLER
FOR SSR OSCILLATIONS DAMPING
In this article optimal control method based on LQR min-
imizes the cost function in order to achieve the optimal
tradeoff between the use of control effort, the magnitude
and the speed of response. Also it guarantees a stable
control system. The Fuzzy logic based on PI-D controller
is implemented to control system in outer loops of con-
troller and designed supplementary controller for damp-
ing oscillation in STATCOM.
It is well known that damping of power system oscil-
lations can be improved by developing a torque in phase
with the speed deviation. Choice of a measurable input
signal is the main consideration in the design of a damp-
ing controller. So for damping purposes, speed deviation
of the generator is used as input to the damping control-
ler and added to the outer dc voltage control loop. This
signal brings to a controller who designed based PI-D
fuzzy controller based on optimal controller as it shown
in Fig.6.
FIGURE 5. PI-D Fuzzy Controller
(12)
FIGURE 6. Proposed Optimal Fuzzy PI-D Supplementary Damping Controller

Zahra Rahimkhani
302 NOVEL FUZZY OPTIMAL CONTROLLER BASED ON STATCOM BIOSCIENCE BIOTECHNOLOGY RESEARCH COMMUNICATIONS
Table 1. Disturbances
Type of Disturbance Time of Occurrence
3 phase fault
Add a Inductive Load
Table 2. Fuzzy controller’s rules
e
e
PB PS ZE NS NB
PB PB PB PS PS ZE
PS PB PS PS ZE NS
ZE PS PS ZE NS NS
NS PS ZE NS NS NB
NB ZE NS NS NB NB
Table 3. Fuzzy controller’s information
Fuzzy controllers
Range
Error Error Deviation Output
Outer dc voltage control
loop
[-1,1] [-0.001,0.001] [-20,20]
Outer reactive power
control loop
[-1,1] [-1,1] [-10,10]
Supplementary damping
controller
[-0.1,-0.1] [-0.001,0.001] [-1,1]
SIMULATION RESULTS
In this paper the case study system is an IEEE benchmark
used to study sub synchronous resonance and particu-
larly torque ampli cation. It consists in a single genera-
tor (600 MVA/22kV/60 Hz/3600 rpm) connected to an
in nite bus via two transmission lines, one of which is
55% series-compensated.
The sub synchronous mode introduced by the compen-
sation capacitor after a three-phase fault has been applied
and cleared excites the oscillatory torsional modes of the
multi-mass shaft and the torque ampli cation phenom-
enon can be observed. The mechanical system is modeled
by 3-masses: mass 1 = generator; mass 2 = low pressure
turbine (LP); mass 3 = high pressure turbine (HP).
Disturbances are accrued in system according table.1.
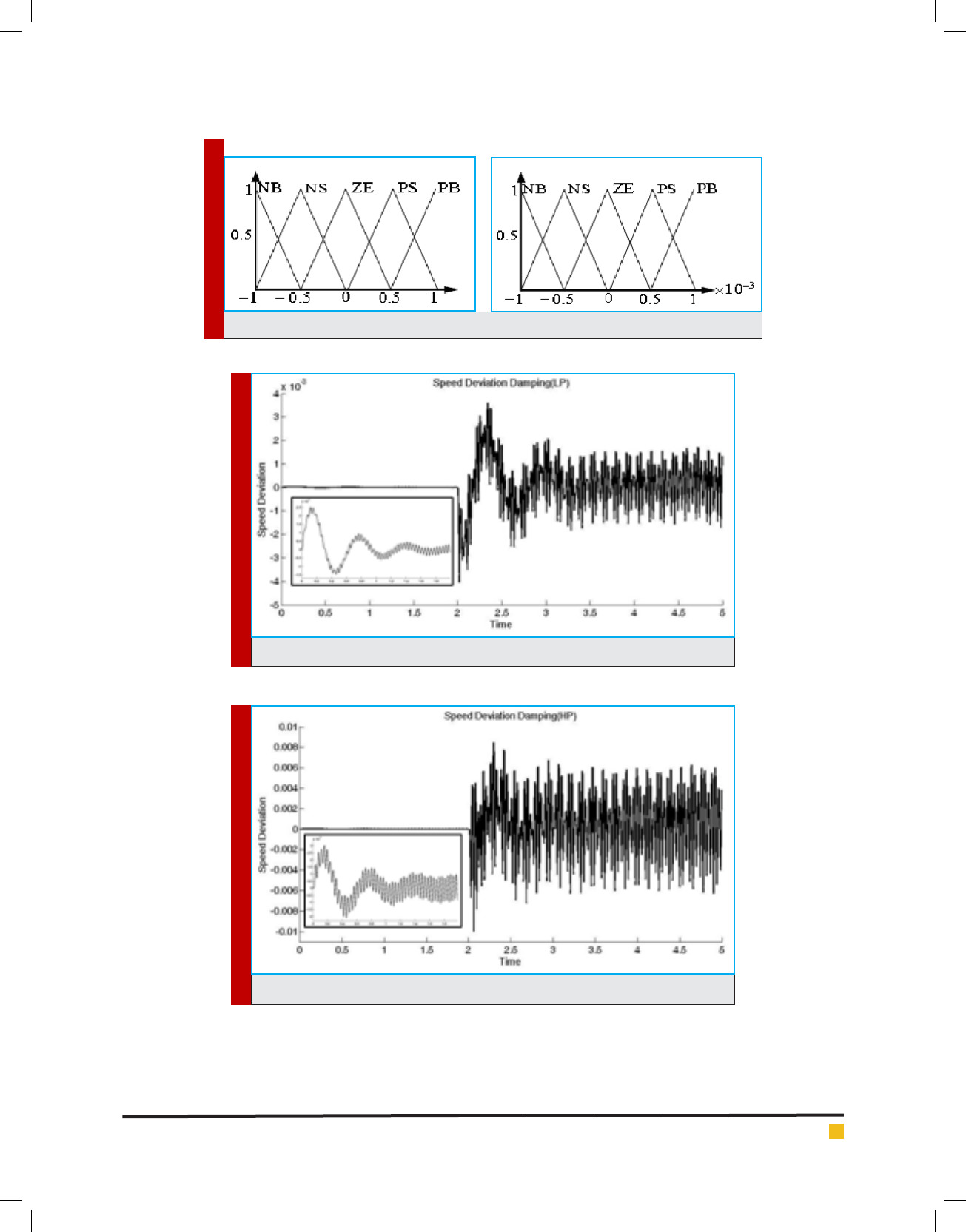
Fig.7 shows the membership functions for fuzzy con-
troller that used in outer dc voltage control loop. Error,
deviation error and controller output range for all fuzzy
controllers are shown in Table.2.
Fuzzy rules that are in fuzzy controllers are shown
in Table.2.
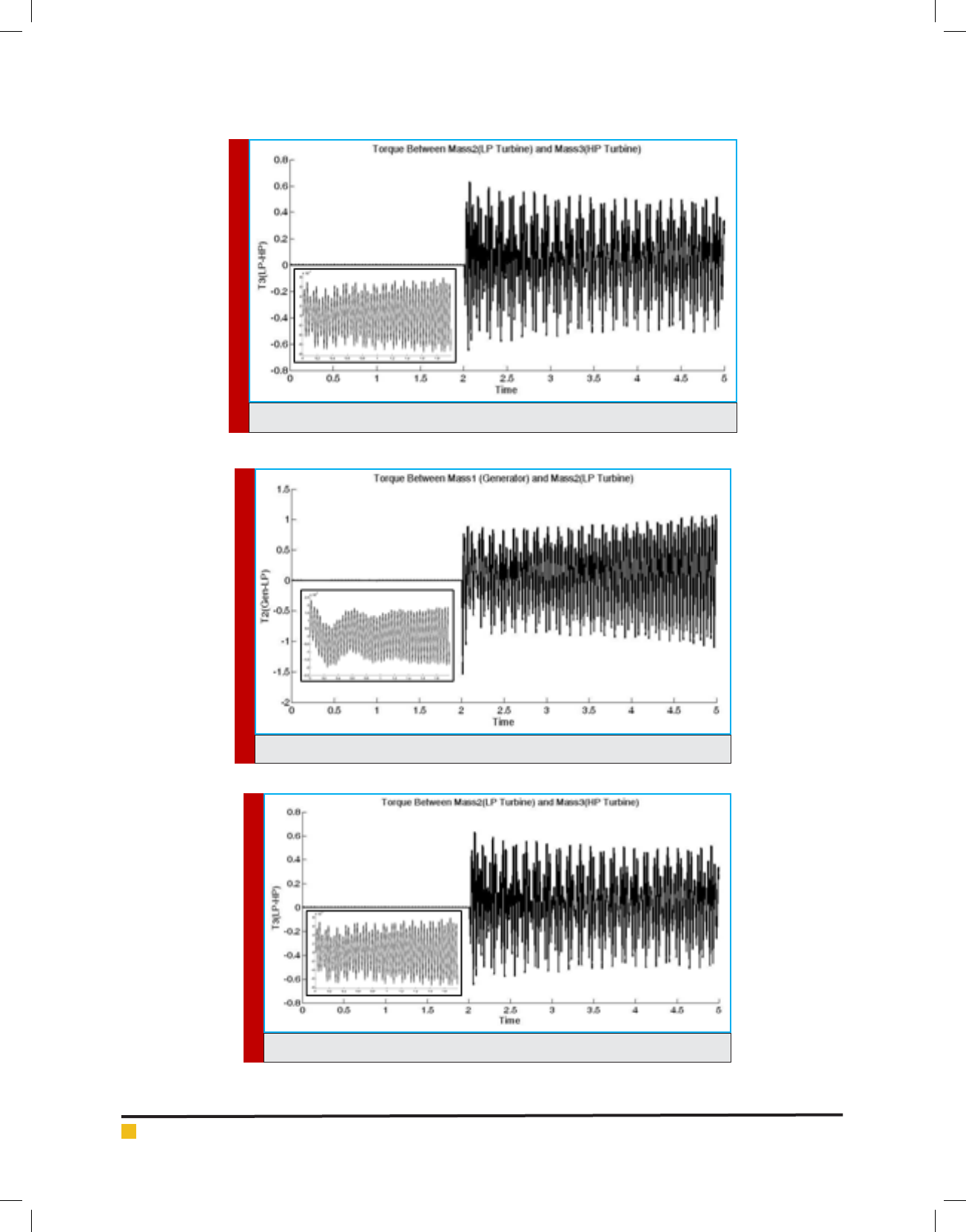
Figures 8-12 describe the response of series compen-
sated system without STATCOM. Figures 8-10 are shown
rotor speed of generator and High and Low pressure tur-
bine shaft speed. Fig. 11 presents torque between gener-
ator and low pressure and Fig. 12 show torque between
generator and high pressure. It is obvious that the com-
pensated system with series capacitive will be unstable
when a 3 phase fault is occurred.
Figures 13-24 are shown system characterizations
after STATCOM installation. Fig. 13 presents the output
voltage of STATCOM which is changed on during the
faults. Fig.14. shows the rotor speed deviation of differ-
ent masses in shaft-rotor system. As it is shown, oscil-
lations in speed are damping during the simulation time
especially when faults are accrued.
Fig.15. represents torque deviation on different
masses. Because of using damping controller in STAT-
COM, torque oscillations are damping. Based on control
strategy in STATCOM, DC voltage of converter must be
constant in all time of simulation. Fig.15. demonstrate
this fact. Figs.16.17. show the three level output volt-
age of converter. Also, voltages of buses are shown
in Fig.17-Fig.21. all voltage has negligible harmonic
because of appropriate lter tuning in output of inverter.
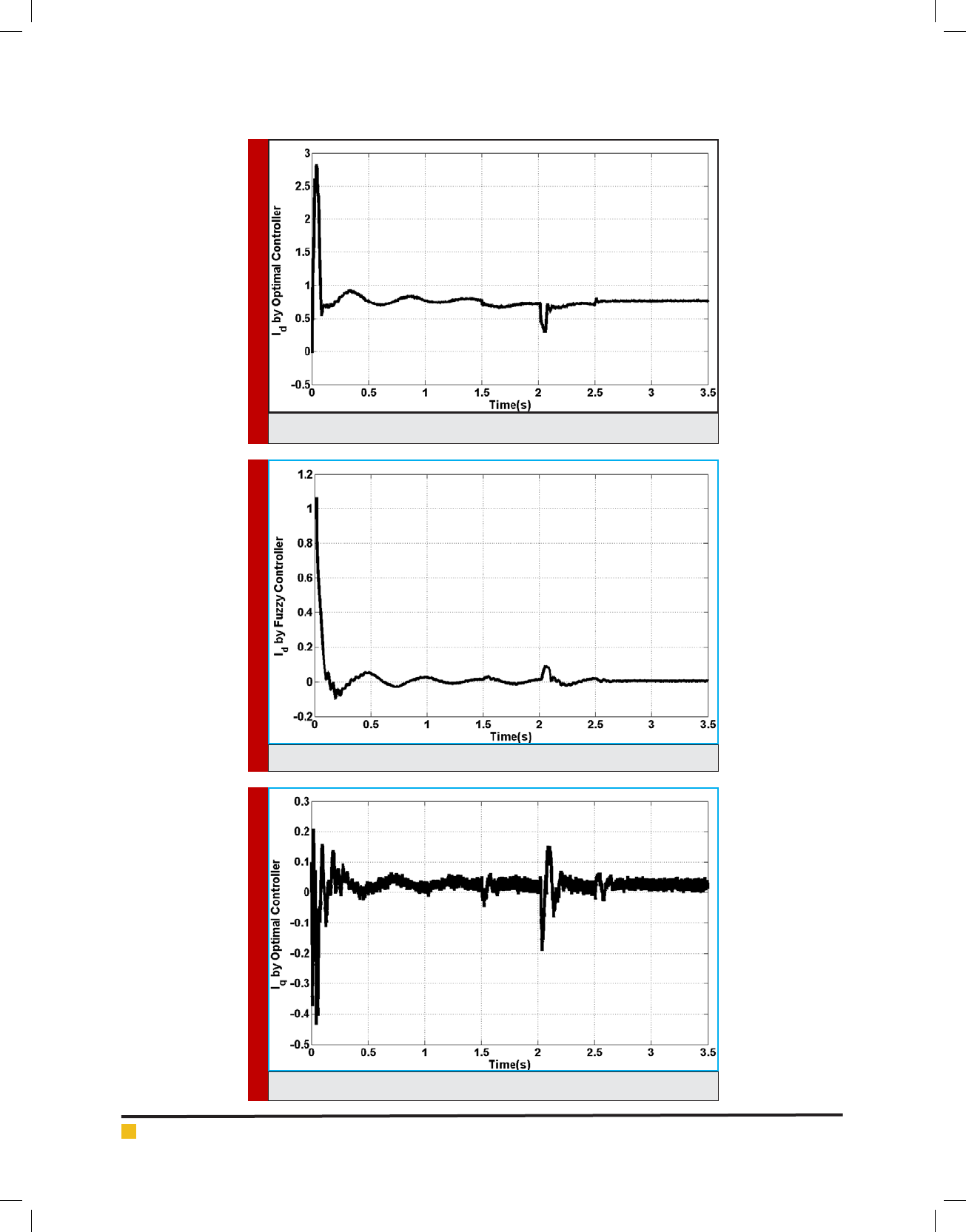
Current components are shown in Fig.22-24. Position of
this current was shown in Fig.6. This is describing pro-
posed technique in this article.
CONCLUSION
In this paper, a novel supplementary subsynchronous
damping controller (SSDC) for STATCOM which is capa-
ble of damping out subsynchronous Resonance (SSR)
oscillations in power system is proposed. This damping
controller is designed based on optimal PI-D fuzzy con-
troller. The presented simulation results show that STAT-
COM based on proposed controller is capable to power
system oscillation damping and. The simulation results
support the applications of optimal fuzzy controller in
power systems.
Zahra Rahimkhani
BIOSCIENCE BIOTECHNOLOGY RESEARCH COMMUNICATIONS NOVEL FUZZY OPTIMAL CONTROLLER BASED ON STATCOM 303
FIGURE 7. Membership functions for outer dc voltage control loop (a) Error(b)Deviation Error
(a)
(b)
FIGURE 8. Generator Speed Deviation
FIGURE 9. Low pressure turbine speed deviation
Zahra Rahimkhani
304 NOVEL FUZZY OPTIMAL CONTROLLER BASED ON STATCOM BIOSCIENCE BIOTECHNOLOGY RESEARCH COMMUNICATIONS
FIGURE 10. High pressure turbine speed deviation
FIGURE 11. Torque between generator and low pressure turbine
FIGURE 12. Torque between low pressure and high pressure turbine

Zahra Rahimkhani
BIOSCIENCE BIOTECHNOLOGY RESEARCH COMMUNICATIONS NOVEL FUZZY OPTIMAL CONTROLLER BASED ON STATCOM 305
FIGURE 13. Output Voltage of STATCOM
FIGURE 14. Rotor Speed Deviation
FIGURE 15. Torque Deviation in Rotor of SM

Zahra Rahimkhani
306 NOVEL FUZZY OPTIMAL CONTROLLER BASED ON STATCOM BIOSCIENCE BIOTECHNOLOGY RESEARCH COMMUNICATIONS
FIGURE 16. Voltage of DC Capacitor in STATCOM
FIGURE 17. Inverter Output Voltage
FIGURE 18. Inverter Output Voltage (0-0.5s)

Zahra Rahimkhani
BIOSCIENCE BIOTECHNOLOGY RESEARCH COMMUNICATIONS NOVEL FUZZY OPTIMAL CONTROLLER BASED ON STATCOM 307
FIGURE 19. Terminal Voltage of SM
FIGURE 20. Terminal Voltage of SG(0-0.5)
FIGURE 21. Middle Bus Voltage
Zahra Rahimkhani
308 NOVEL FUZZY OPTIMAL CONTROLLER BASED ON STATCOM BIOSCIENCE BIOTECHNOLOGY RESEARCH COMMUNICATIONS
FIGURE 22. Active Component of LQR Controller
FIGURE 23. Active Component of Fuzzy Controller
FIGURE 24. Reactive Component of LQR Controller

Zahra Rahimkhani
BIOSCIENCE BIOTECHNOLOGY RESEARCH COMMUNICATIONS NOVEL FUZZY OPTIMAL CONTROLLER BASED ON STATCOM 309
FIGURE 25. Reactive Component of LQR Controller
REFERENCES
Zhu,X., H.Sun, J.Wen, S.Chen (2014), A practical method to
construct network state equations in multi-machine system
SSR study”, Electric Power Systems Research 107, pp 51– 58.
Bo, W Z. Yan (2002), Damping subsynchronous oscillation
usingUPFC—aFACTS device, in Proc. Int. Conf. Power Sys-
tem Technology, vol. 4—PowerCon 2002, October 13–17, pp.
2298–2301.
Bongiorno, M., L.Ängquist, J.S.Lennart (2008), “A novel con-
trol strategy for subsynchronous resonance mitigation using
SSSC”, IEEE Trans. Power Electron, pp 1033–10041.
Bongiorno, M., J. Svensson, L. Ängquist (2008), “On control
of static synchronous series compensator for SSR mitigation”,
IEEE Trans. Power Electron, pp 735–743.
Edris A(1990), Series compensation schemes reducing the
potential of resonance, IEEE Trans PAS, pp219–26.
Fouad AA (1978), Khu KT. Subsynchronous resonance zones
in the IEEE bench mark power system IEEE Trans PAS, PAS-
97(15), pp 754–62.
Framer RG, Katz E, Schwalb AL. Navajo (1985), Project on sub
synchronous resonance analysis and solutions IEEE Trans PAS,
pp1057–66.
IEEE SSR Task Force, First benchmark model for computer
simulation of sub synchronous resonance, IEEE Trans. Power
Appl. Syst. PAS-96 (September/October) 1562–1572.
IEEE SSR Working Group (1985), Terms, de nitions and sym-
bols for sub synchronous oscillations, IEEE Trans. Power Appl.
Syst. PAS-104 (June (6)), pp1326–1334.
IEEE Sub synchronous Resonance working group, Second
benchmark model for computer simulation of sub synchro-
nous resonance, IEEE Transactions on Power Apparatus and
Systems, vol. PAS-104, no. 5, 1985, pp. 1057-1066.
Iravani MR, Edris AA (1994), “Eigen analysis of series com-
pensation schemes reducing the potential of sub synchronous
resonance”. IEEE Power System Summer Meeting.
Jang JSR, C.T. Sun & E (2010). Mizutani: Neuro Fuzzy and Soft
Computing”, PHI Learning.
Li Yidan, Lu Wensheng, Peng Xiuyan (2011), DC voltage meas-
urement and control for cascaded STATCOM”, Proceedings of
the CSEE, Vol. 1, Issue 31, pp. 14-19.
Liu X Single (2013), Neuron Self-tuning PID Control for
Welding Molten Pool Depth, Proceedings of the 7 Th World
Congress on Intelligent Control and AutomationJune 25-27,
Chongqing, China
Mon, YJ C.M. Lin & I.J. Rudas (2013), ANFIS-based Wireless
Sensor Network (WSN) Applications for Air Conditioner Con-
trol, Acta Polytechnica Hungarica, Vol. 10, No. 3, pp. 5-16
Orman,M., P.Balcerek, M.Orkisz (2012), Effective method of
subsynchronous resonance detection and its limitations”, Elec-
trical Power and Energy Systems 43, pp915–920
Padiyar, K., N.Prabhu (2006), Design and performance evalua-
tion of sub synchronous damping controller with STATCOM”,
IEEE Trans. Power Deliv. 21 (July (3)), pp1398–1405.
Pahlavani, M., H.Mohamadpour (2011), Damping of sub-syn-
chronous resonance and low-frequency power oscillation in
a series-compensated transmission line using gate-controlled
series capacitor”, Electric Power Systems Research 81, pp 308–
317.
Pilotto, LAS A. Bianco, W.F. Long (2003), A.A. Edris, Impact of
TCSC control methodologies on subsynchronous oscillations,
IEEE Trans. Power Deliv, pp 243–252.
Quanyuan Y. Jiang, Yijia J. Cao and Shijie J. Cheng (2005), A
Genetic Approach to Design a HVDC Supplementary Subsyn-
chronous Damping Controller”, IEEE Transactions On Power
Delivery, Vol. 20, NO.2.

Zahra Rahimkhani
310 NOVEL FUZZY OPTIMAL CONTROLLER BASED ON STATCOM BIOSCIENCE BIOTECHNOLOGY RESEARCH COMMUNICATIONS
Rana RD, Huff SW, Hayes RM, Fromholtz EN, Schulz RP (2009),
AEP’s Kanawha river 345kV series capacitor installations-sub-
synchronous resonance studies and torsional measurements”,
In Proceedings of the American power conference, p 300–5.
Wei Wenhui, Liu Wenhua, Song Qiang (2005), Research on fast
dynamic control of static synchronous compensator using cas-
cade multilevel inverters”, Proceedings of the CSEE, Vol. 25,
Issue 2, pp. 23-26.
Xie, XR Y.P. Dong, Y.D. Han (2012), “Online estimation of turbine–
generator shaft fatigue loss-of-life caused by sub synchronous
resonance”, Electric Power Systems Research 92, pp 171–179.
Xin, L.Shao Wenchang, Yang Shuying (2009), Multi- loop
control scheme of grid side converter with LCL lter, Journal
of Hefei University of Technology, Vol. 7, Issue 32, pp 972-
976.
Yan A, Yu Y (1982), Multi-mode stabilization of torsional
oscillations using output feedback excitation control, IEEE
Trans PAS, PAS-101, pp 1245–53.
Zhu W, Spee R, Mohler RR, Alexander GG, Mittelstadt WA,
Maratuhulam D (1995), “An EMTP study of SSR mitigation
using the thyristor controlled series capacitor”, IEEE Trans
Power, pp 10:1479–85.